This information is part of the Modelica Standard Library maintained by the Modelica Association.

Calculation of the mean convective heat transfer coefficient kc for a helical pipe in the laminar flow regime.

Functions kc_laminar and kc_laminar_KC

There are basically three differences:

• The function kc_laminar is using kc_laminar_KC but offers additional output variables like e.g. Reynolds number or Nusselt number and failure status (an output of 1 means that the function is not valid for the inputs).
• Generally the function kc_laminar_KC is numerically best used for the calculation of the mean convective heat transfer coefficient kc at known mass flow rate.
• You can perform an inverse calculation from kc_laminar_KC, where an unknown mass flow rate is calculated out of a given mean convective heat transfer coefficient kc

Restriction

• laminar regime (Reynolds number ≤ critical Reynolds number Re_crit)
• neglect influence of heat transfer direction (heating/cooling) according to Sieder and Tate

The critical Reynolds number Re_crit in a helical pipe depends on its mean curvature diameter d_coil . The smaller the mean curvature diameter of the helical pipe, the earlier the turbulent regime will start due to vortexes out of higher centrifugal forces.

Geometry

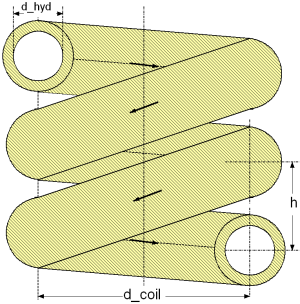
Calculation

The mean convective heat transfer coefficient kc for helical pipes is calculated through the corresponding Nusselt number Nu according to [VDI 2002, p. Gc 2, eq. 5] :

    Nu = 3.66 + 0.08*[1 + 0.8*(d_hyd/d_coil)^0.9]*Re^m*Pr^(1/3)

with the exponent m for the Reynolds number

    m = 0.5 + 0.2903*(d_hyd/d_coil)^0.194

and the resulting mean convective heat transfer coefficient kc

    kc =  Nu * lambda / d_hyd

with

d_mean	as mean diameter of helical pipe [m],
d_coil = f(geometry)	as mean curvature diameter of helical pipe [m],
d_hyd	as hydraulic diameter of the helical pipe [m],
h	as slope of helical pipe [m],
kc	as mean convective heat transfer coefficient [W/(m2K)],
lambda	as heat conductivity of fluid [W/(mK)],
L	as total length of helical pipe [m],
Nu = kc*d_hyd/lambda	as mean Nusselt number [-],
Pr = eta*cp/lambda	as Prandtl number [-],
Re = rho*v*d_hyd/eta	as Reynolds number [-],
Re_crit = f(geometry)	as critical Reynolds number [-].

Verification

The mean Nusselt number Nu representing the mean convective heat transfer coefficient kc is shown below for different numbers of turns n_nt at constant total length of the helical pipe.

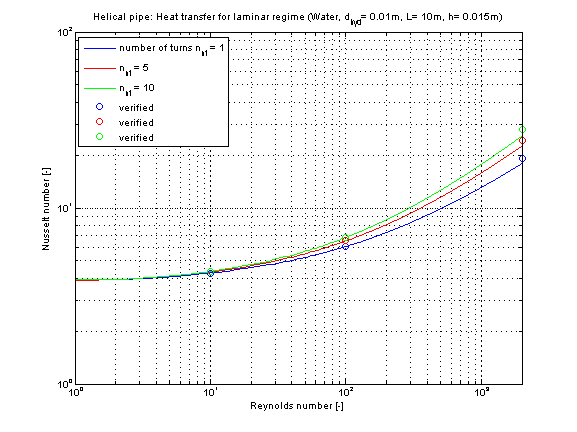
The convective heat transfer of a helical pipe is enhanced compared to a straight pipe due to occurring turbulences resulting out of centrifugal forces. The higher the number of turns, the better is the convective heat transfer for the same length of a pipe.

Note that the ratio of hydraulic diameter to total length of helical pipe d_hyd/L has no remarkable influence on the coefficient of heat transfer kc .

References

GNIELINSKI, V.:

Heat transfer and pressure drop in helically coiled tubes.. In 8th International Heat Transfer Conference, volume 6, pages 2847-2854, Washington,1986. Hemisphere.