This information is part of the Modelica Standard Library maintained by the Modelica Association.

Restriction

This function shall be used within the restricted limits according to the referenced literature.

• Smooth surface
• Turbulent flow regime
• Reynolds number for sudden expansion Re > 3.3e3 [Idelchik 2006, p. 208, diag. 4-1]
• Reynolds number for sudden contraction Re > 1e4 [Idelchik 2006, p. 216-217, diag. 4-9]

Geometry

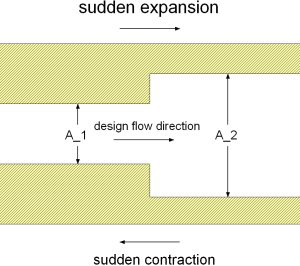
Calculation

The local pressure loss dp is generally determined by:

    dp = 0.5 * zeta_LOC * rho * |v_1|*v_1

with

rho	as density of fluid [kg/m3],
v_1	as average flow velocity in small cross sectional area [m/s].
zeta_LOC	as local resistance coefficient [-],

The local resistance coefficient zeta_LOC of a sudden expansion can be calculated for different ratios of cross sectional areas by:

    zeta_LOC = (1 - A_1/A_2)^2  [Idelchik 2006, p. 208, diag. 4-1] 

and for sudden contraction:

    zeta_LOC = 0.5*(1 - A_1/A_2)^0.75  [Idelchik 2006, p. 216-217, diag. 4-9] 

with

A_1	small cross sectional area [m^2],
A_2	large cross sectional area [m^2].

Verification

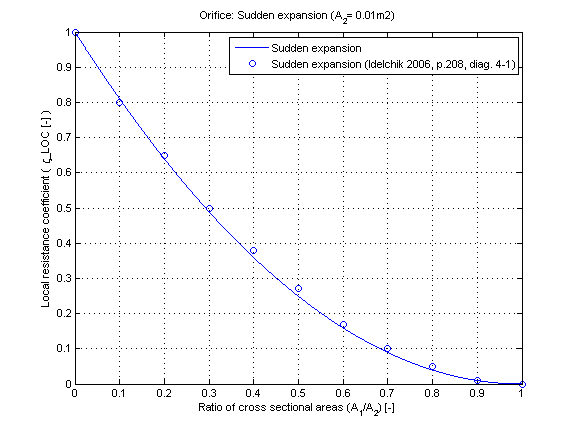
The local resistance coefficient zeta_LOC of a sudden expansion in dependence of the cross sectional area ratio A_1/A_2 is shown in the figure below.

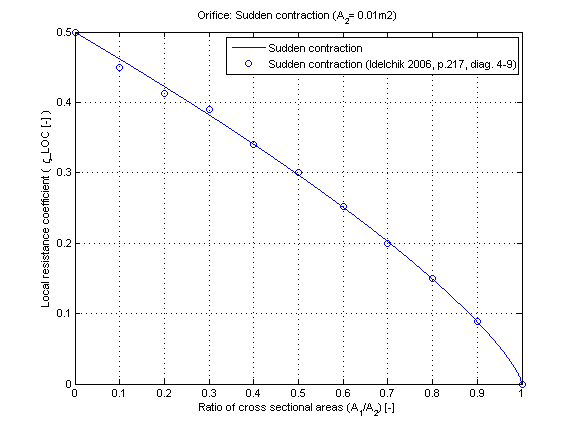
The local resistance coefficient zeta_LOC of a sudden contraction in dependence of the cross sectional area ratio A_1/A_2 is shown in the figure below.

References

Elmqvist, H., M.Otter and S.E. Cellier:

Inline integration: A new mixed symbolic / numeric approach for solving differential-algebraic equation systems.. In Proceedings of European Simulation MultiConference, Praque, 1995.

Idelchik,I.E.:

Handbook of hydraulic resistance. Jaico Publishing House, Mumbai, 3rd edition, 2006.